| Home | Registration | Program | Talks | Practical Information |
Several areas of mathematics provide useful tools for the study of quivers and associative algebras. Through three lecture series by experts and contributed talks by participants, this summer school will explore novel categorical and geometrical innovations in the field.
The school is primarily aimed towards junior participants, i.e., Ph.D. students and postdocs. In order to encourage more active participation by students, the first two talks of each mini-course along with the research talks are intended to be given by them. If you wish to give a talk, please find more information, along with general information for registration, here.
To download the poster, please click on the image below.
|
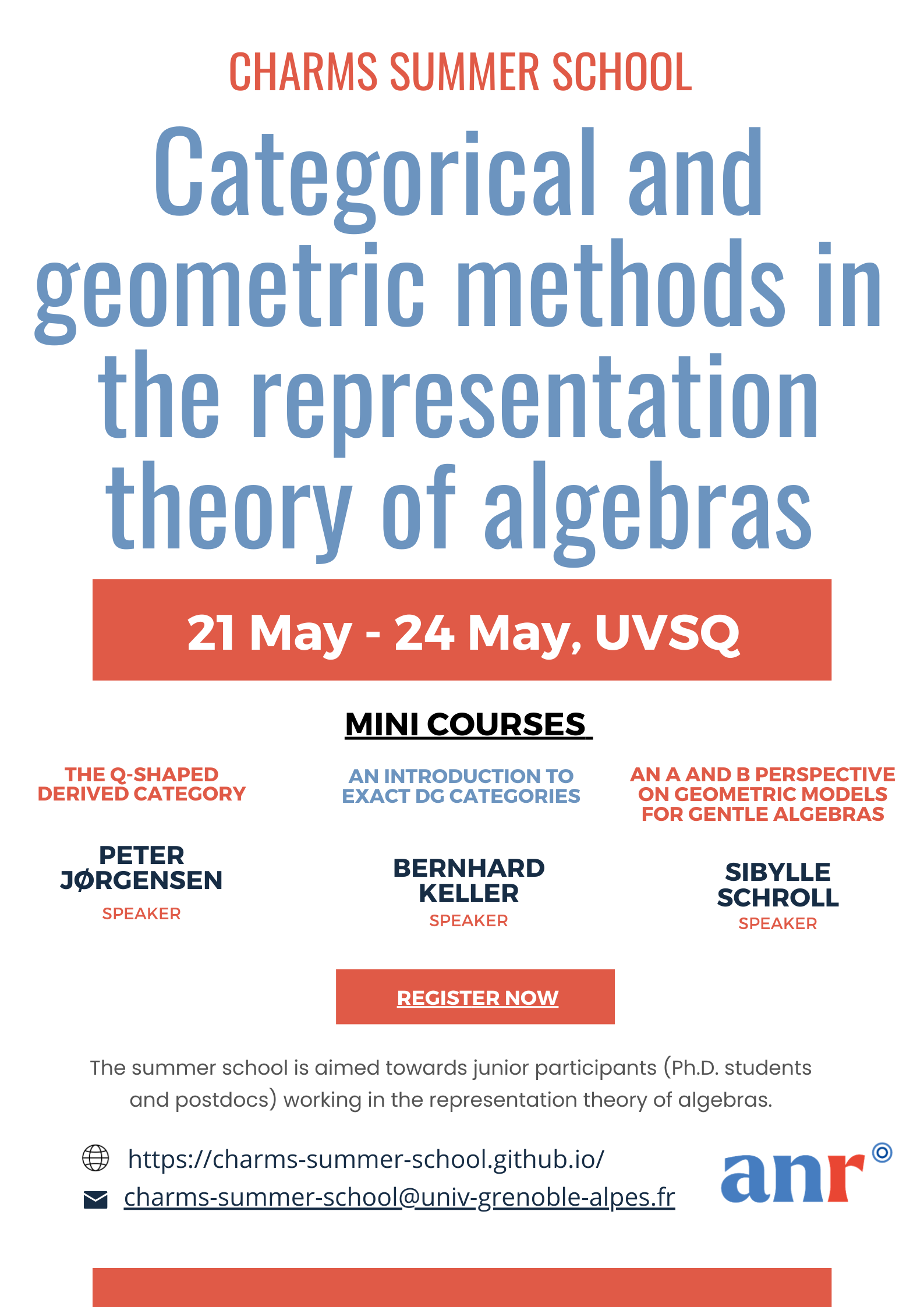
This minicourse is an introduction to exact dg categories following the work of Xiaofa Chen in his Ph. D. thesis and several more recent preprints. Chen's notion of exact dg category is a simultaneous generalization of the notions of exact category in the sense of Quillen and of pretriangulated dg category in the sense of Bondal-Kapranov. The first two lectures will be devoted to these predecessors. We will then give a definition of exact dg category in complete analogy with Quillen's but where the category of kernel-cokernel pairs is replaced with a more sophisticated homotopy category. There will follow a number of fundamental results concerning the dg nerve, the dg derived category, tensor products and functor categories with exact dg target. In the final lectures, we will give applications of the framework of exact dg categories in the study of lower and higher Auslander correspondence and some of its generalizations.
In this lecture series we begin by introducing the class of gentle algebras. Gentle algebras are associative algebras given by quiver and relations originally arising as iterated tilted algebras of type A. While intensely studied in representation theory, it is remarkable that gentle algebras appear in many different contexts. One such context is homologically mirror symmetry related to surfaces with stops. While the majority of the lectures will be concerned with the A-side, that is the relation of gentle algebras to partially wrapped Fukaya categories of surfaces with stops, we will begin the lecture series with an introduction to gentle algebras and their representation theory by Kyoungmo Kim and an introduction to what is known of the relations of gentle algebras to the B-side of homological mirror symmetry, notably in the work of Burban and Drozd (and also Lekili and Polishchuk) presented by Kyungmin Rho.
The classic derived category \(\mathscr{D}\) can be viewed as a category of representations of a certain quiver with relations, \(Q^{\mathrm{cpx}}\). The vertices of \(Q^{\mathrm{cpx}}\) are the integers, there is an arrow \(q \rightarrow{} q-1\) for each integer \(q\), and the relations are that consecutive arrows compose to \(0\). The representations of \(Q^{\mathrm{cpx}}\) are precisely the chain complexes, that is, the objects of \(\mathscr{D}\).
Many good properties of \(\mathscr{D}\) rely on \(Q^{\mathrm{cpx}}\) having a Serre functor when viewed as a small category. Generalising the construction of \(\mathscr{D}\) to other quivers with relations which have a Serre functor results in the \(Q\)-shaped derived category, \(\mathscr{D}_Q\).
The lectures are an introduction to the definition and basic properties of \(\mathscr{D}_Q\), which has the derived category of \(N\)-complexes and the periodic derived category as special cases.
This summer school is organized by junior faculty with links to CHARMS. The organizing committee consists of:
In case of any queries, please contact the organizers by writing to charms-summer-school@univ-grenoble-alpes.fr.
We gratefully acknowledge the funding by our sponsors.
|
|
|
|
|
|
|